Skip to main content
SpringerLink
Log in

Existence of Single-Peak Solitary Waves and Double-Peaks Solitary Wave of Gardner Equation with Kuramoto–Sivashinsky Perturbation

  • Published:
Qualitative Theory of Dynamical Systems Aims and scope Submit manuscript

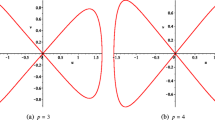
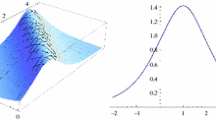
Abstract

This paper concerns geometric study of single-peak solitary waves and double-peaks solitary wave of Gardner equation with Kuramoto–Sivashinsky perturbation. We first reduce the high-dimensional traveling wave system of the perturbed Gardner equation to the perturbed planar system through geometric singular perturbation theory. We then show the persistence of one homoclinic orbit, and the generation of a new homoclinic orbit by the Melnikov function method. Single-peak solitary waves and double-peaks solitary wave are newly found for the perturbed Gardner equation. The numerical simulations are performed to verify the theoretical results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  1. Ogawa, T.: Travelling wave solutions to a perturbed Korteweg-de Vries equation. Hiroshima Math. J. 24(2), 401–422 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  2. Yan, W., Liu, Z., Liang, Y.: Existence of solitary waves and periodic waves to a perturbed generalized KdV equation. Math. Modell. Anal. 19(4), 537–555 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  3. Chen, A., Guo, L., Deng, X.: Existence of solitary waves and periodic waves for a perturbed generalized BBM equation. J. Differ. Equ. 261(10), 5324–5349 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  4. Wen, Z., Zhang, L., Zhang, M.: Dynamics of classical Poisson–Nernst–Planck systems with multiple cations and boundary layers. J. Dyn. Differ. Equ. 33(1), 211–234 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  5. Ge, J., Du, Z.: The solitary wave solutions of the nonlinear perturbed shallow water wave model. Appl. Math. Lett. 103, 106202 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  6. Guo, L., Zhao, Y.: Existence of periodic waves for a perturbed quintic BBM equation. Discr. Contin. Dyn. Syst. 40(8), 4689 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  7. Wen, Z.: On existence of kink and antikink wave solutions of singularly perturbed Gardner equation. Math. Methods Appl. Sci. 43(7), 4422–4427 (2020)

    MathSciNet  MATH  Google Scholar 

  8. Zhang, H., Xia, Y., N’gbo, P.: Global existence and uniqueness of a periodic wave solution of the generalized Burgers-Fisher equation. Appl. Math. Lett. 121, 107353 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  9. Wen, Z., Bates, P.W., Zhang, M.: Effects on I–V relations from small permanent charge and channel geometry via classical Poisson–Nernst–Planck equations with multiple cations. Nonlinearity 34(6), 4464 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  10. Zhang, L., Han, M., Zhang, M., Khalique, C.M.: A new type of solitary wave solution of the mKdV equation under singular perturbations. Int. J. Bifurc. Chaos 30(11), 2050162 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  11. Sun, X., Huang, W., Cai, J.: Coexistence of the solitary and periodic waves in convecting shallow water fluid. Nonlinear Anal. Real World Appl. 53, 103067 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  12. Huang, Z., Wen, Z.: Single-and double-peak solitary waves of two-component Drinfel’d–Sokolov–Wilson system with Kuramoto–Sivashinsky perturbation. Int. J. Bifurc. Chaos 33(01), 2350007 (2023)

    Article  MathSciNet  Google Scholar 

  13. Bates, P.W., Wen, Z., Zhang, M.: Small permanent charge effects on individual fluxes via Poisson-Nernst-Planck models with multiple cations. J. Nonlinear Sci. 31(3), 55 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  14. Kamchatnov, A., Kuo, Y., Lin, T., Horng, T., Gou, S., Clift, R., El, G., Grimshaw, R.: Undular bore theory for the Gardner equation. Phys. Rev. E 86(3), 036605 (2012)

    Article  Google Scholar 

  15. Miura, R.M.: Korteweg-de Vries equation and generalizations. I. A remarkable explicit nonlinear transformation. J. Math. Phys. 9(8), 1202–1204 (1968)

    Article  MathSciNet  MATH  Google Scholar 

  16. Miura, R.M., Gardner, C.S., Kruskal, M.D.: Korteweg-de Vries equation and generalizations. II. Existence of conservation laws and constants of motion. J. Math. Phys. 9(8), 1204–1209 (1968)

    Article  MathSciNet  MATH  Google Scholar 

  17. Watanabe, S.: Ion acoustic soliton in plasma with negative ion. J. Phys. Soc. Jpn. 53(3), 950–956 (1984)

    Article  Google Scholar 

  18. Ruderman, M.S., Talipova, T., Pelinovsky, E.: Dynamics of modulationally unstable ion-acoustic wavepackets in plasmas with negative ions. J. Plasma Phys. 74(5), 639–656 (2008)

    Article  Google Scholar 

  19. Grimshaw, R.: Environmental Stratified Flows, no. 3. Springer Science & Business Media, Berlin (2002)

  20. Grimshaw, R., Pelinovsky, E., Taipova, T., Sergeeva, A.: Rogue internal waves in the ocean: long wave model. Eur. Phys. J. Special Top. 185(1), 195–208 (2010)

    Article  Google Scholar 

  21. Demler, E., Maltsev, A.: Semiclassical solitons in strongly correlated systems of ultracold bosonic atoms in optical lattices. Ann. Phys. 326(7), 1775–1805 (2011)

    Article  MATH  Google Scholar 

  22. Alejo, M.A.: Nonlinear stability of Gardner breathers. J. Differ. Equ. 264(2), 1192–1230 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  23. Grimshaw, R., Slunyaev, A., Pelinovsky, E.: Generation of solitons and breathers in the extended Korteweg-de Vries equation with positive cubic nonlinearity. Chaos 20(1), 013102 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  24. Fu, Z., Liu, S., Liu, S.: New kinds of solutions to Gardner equation. Chaos Solitons Fractals 20(2), 301–309 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  25. Wazwaz, A.M.: New solitons and kink solutions for the Gardner equation. Commun. Nonlinear Sci. Numer. Simul. 12(8), 1395–1404 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  26. Betchewe, G., Victor, K.K., Thomas, B.B., Crepin, K.T.: New solutions of the Gardner equation: analytical and numerical analysis of its dynamical understanding. Appl. Math. Comput. 223, 377–388 (2013)

    MathSciNet  MATH  Google Scholar 

  27. Saha, A., Talukdar, B., Chatterjee, S.: Dynamical systems theory for the Gardner equation. Phys. Rev. E 89(2), 023204 (2014)

    Article  Google Scholar 

  28. Chen, Y., Liu, Z.: The bifurcations of solitary and kink waves described by the Gardner equation. Discr. Contin. Dyn. Syst. S 9(6), 1629–1645 (2016)

    Article  MathSciNet  MATH  Google Scholar 

  29. Fei, J., Cao, W., Ma, Z.: Nonlocal symmetries and explicit solutions for the Gardner equation. Appl. Math. Comput. 314, 293–298 (2017)

    MathSciNet  MATH  Google Scholar 

  30. Ak, T., Triki, H., Dhawan, S., Erduran, K.S.: Theoretical and numerical investigations on solitary wave solutions of Gardner equation. Eur. Phys. J. Plus 133(9), 382 (2018)

    Article  Google Scholar 

  31. Hepson, O.E., Korkmaz, A., Dag, I.: Exponential B-spline collocation solutions to the Gardner equation. Int. J. Comput. Math. 97(4), 837–850 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  32. Slyunyaev, A., Pelinovski, E.: Dynamics of large-amplitude solitons. J. Exp. Theor. Phys. 89(1), 173–181 (1999)

    Article  Google Scholar 

  33. Tang, Y., Xu, W., Shen, J., Gao, L.: Persistence of solitary wave solutions of singularly perturbed Gardner equation. Chaos Solitons Fractals 37(2), 532–538 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  34. Fenichel, N.: Geometric singular perturbation theory for ordinary differential equations. J. Differ. Equ. 31(1), 53–98 (1979)

    Article  MathSciNet  MATH  Google Scholar 

  35. Han, M.: Bifurcation Theory of Limit Cycles. Science Press, Beijing (2013)

    Google Scholar 

Download references

Acknowledgements

This work is partially supported by the National Natural Science Foundation of China (12071162), the Natural Science Foundation of Fujian Province (No. 2021J01302) and the Fundamental Research Funds for the Central Universities (No. ZQN-802).

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Huaqiao University, Quanzhou, 362021, People’s Republic of China

    Keqin Zhao & Zhenshu Wen

Authors
  1. Keqin Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Zhenshu Wen
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

KZ carried out the analytical studies and wrote the draft paper. ZW conceived the study and the overall manuscript design, and reviewed and revised the paper. All authors read and approved the final manuscript.

Corresponding author

Correspondence to Zhenshu Wen.

Ethics declarations

Conflict of interest

The authors declare no competing interests.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhao, K., Wen, Z. Existence of Single-Peak Solitary Waves and Double-Peaks Solitary Wave of Gardner Equation with Kuramoto–Sivashinsky Perturbation. Qual. Theory Dyn. Syst. 22, 112 (2023). https://doi.org/10.1007/s12346-023-00811-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12346-023-00811-1

Keywords

Search

Navigation