Skip to main content
SpringerLink
Log in

On wall-crossing invariance of certain sums of Welschinger numbers

  • Published:
Selecta Mathematica Aims and scope Submit manuscript

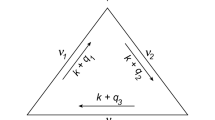
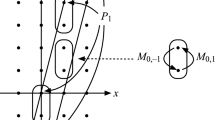
The obvious answer is always overlooked.

Known as Whitehead’s Law.

Abstract

We continue our quest for real enumerative invariants not sensitive to changing the real structure and extend the construction we uncovered previously for counting curves of anti-canonical degree \(\leqslant 2\) on del Pezzo surfaces with \(K^2=1\) to curves of any anti-canonical degree and on any del Pezzo surfaces of degree \(K^2\leqslant 3\).

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Notes

  1. In fact, this theorem, with appropriate definitions for the numbers \(\Gamma _{B,k}\), holds for all real rational surfaces X with any \({\text {Pin}}^-\)-structure on \(X_\mathbb R\).

  2. Properties (4) and (5) hold without any assumption on a real structure of X.

References

  1. Bourbaki, N.: Groupes et Algèbres de Lie, Ch. 4, 5 et 6. Hermann, Paris (1968)

  2. Brugallé, E.: On the invariance of Welschinger invariants. Algebra i Analiz 32, 1–20 (2020)

    MathSciNet  Google Scholar 

  3. Brugallé, E., Puignau, N.: On Welschinger invariants of symplectic 4-manifolds. Comment. Math. Helv. 90, 905–938 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  4. Chen, X.: Steenrod pseudocycles, lifted cobordisms, and Solomon’s relations for Welschinger’s invariants. Geom. Funct. Anal. 32(3), 490–567 (2022)

  5. Chen, X., Zinger, A.: WDVV-type relations for Welschinger invariants: applications. Kyoto J. Math. 61, 339–376 (2021)

    Article  MathSciNet  MATH  Google Scholar 

  6. Comtet, L.: Advanced Combinatorics: The Art of Finite and Infinite Expansions. D. Reidel Publishing Company, Dordrecht (1974)

    Book  MATH  Google Scholar 

  7. Corless, R.M., Gonnet, G.H., Hare, D.E.G., Jeffrey, D.J., Knuth, D.E.: On the Lambert W function. Adv. Comput. Math. 5, 329–359 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  8. Degtyarev, A., Itenberg, I., Kharlamov, V.: Real Enriques Surfaces. Springer, Lecture Notes in Mathematics 1746 (2000)

  9. Degtyarev, A.I., Kharlamov, V.M.: Topological properties of real algebraic varieties: Rokhlin’s way. Russ. Math. Surv. 55(4), 735–814 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  10. Dolgachev, I.V.: Classical Algebraic Geometry. A Modern View. Cambridge University Press, Cambridge (2012)

    Book  MATH  Google Scholar 

  11. Finashin, S., Kharlamov, V.: Abundance of real lines on real projective hypersurfaces. Int. Math. Res. Not. IMRN (2013). https://doi.org/10.1093/imrn/rns135

    Article  MathSciNet  MATH  Google Scholar 

  12. Finashin, S., Kharlamov, V.: Two kinds of real lines on real del Pezzo surfaces of degree 1. Selecta Math. (N.S.) (2021). https://doi.org/10.1007/s00029-021-00690-x

    Article  MathSciNet  MATH  Google Scholar 

  13. Finashin, S., Kharlamov, V.: Combined count of real rational curves of canonical degree 2 on real del Pezzo surfaces with \(K^2=1\). J. Inst Math Jussieu. pp. 1–26. https://doi.org/10.1017/S1474748022000317

  14. Horev, A., Solomon, J.P.: The open Gromov–Witten–Welschinger theory of blowups of projective plane. arXiv:1210.4034, 34 pp

  15. Itenberg, I., Shustin, E., Kharlamov, V.: Welschinger invariants of small non-toric Del Pezzo surfaces. J. Eur. Math. Soc. (JEMS) 15(2), 539–594 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  16. Itenberg, I., Shustin, E., Kharlamov, V.: Welschinger invariants of real del Pezzo surfaces of degree \(\ge 2\). Int. J. Math. 26(6), 1550060 (2015) 63 pp

  17. Itenberg, I., Shustin, E., Kharlamov, V.: Welschinger invariants revisited. In: Analysis Meets Geometry: A Tribute to Mikael Passare. Trends in Mathematics, Springer. pp. 239 – 260 (2017)

  18. Kirby, R., Taylor, L.: Pin-structures on low-dimensional manifolds. In: Donaldson, S. K., Thomas, C. B. (eds) Geometry of Low-dimensional Manifolds, 2. London Mathematical Society Lecture Note (151), Cambridge University Press (1991)

  19. Kontsevich, M., Manin, Yu.: Gromov-Witten classes, quantum cohomology, and enumerative geometry. Commun. Math. Phys. 164, 525–562 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  20. Moon, J.W.: Counting labelled trees. Canadian Mathematical Monographs 1 (1970) 113 pp

  21. Nikulin, V.V.: Integer symmetric bilinear forms and some of their geometric applications. Izv. Akad. Nauk SSSR Ser. Mat. 43(1), 111–177 (1979)

    MathSciNet  MATH  Google Scholar 

  22. Okonek, C., Teleman, A.: Intrinsic signs and lower bounds in real algebraic geometry. J. Reine Ang. Math. 688, 219–241 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  23. Solomon, J.P.: Intersection theory on the moduli space of holomorphic curves with Lagrangian boundary conditions. arXiv:math/0606429 (2006) 79 pp

  24. Solomon, J.P.: A differential equation for the open Gromov–Witten potential. (2007, preprint)

  25. Tehrani, F.: Open Gromov-Witten theory on symplectic manifolds and symplectic cutting. Adv. Math. 232, 238–270 (2013)

    Article  MathSciNet  MATH  Google Scholar 

  26. Walcher, J.: Opening mirror symmetry on the quintic. Commun. Math. Phys. 276(3), 671–689 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  27. Welschinger, J.-Y.: Invariants of real symplectic 4-manifolds and lower bounds in real enumerative geometry. Invent. Math. 162(1), 195–234 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  28. Welschinger, J.-Y.: Optimalité, congruences et calculs d’invariants des variétés symplectiques réelles de dimension quatre. arXiv:0707.4317

Download references

Acknowledgements

The idea of this work arose during a stay of the second author at the Max-Planck Institute for Mathematics in Bonn in spring 2021 and took its shape during a RIP-stay of the authors at the Mathematisches Forschungsinstitut Oberwolfach in summer 2021. We thank the both institutions for hospitality and excellent (despite a complicated pandemic situation) working conditions. Our special thanks go to R. Rasdeaconu, discussions with whom were among the motivations for this study, and to J. Solomon, for encouragement and helpful remarks. We also thankful to the referee for careful reading and valuable comments. The second author was partially supported by the grant ANR-18-CE40-0009 of French Agence Nationale de Recherche and the grant by Ministry of Science and Higher Education of Russia under the contract 075-15-2019-1620 with St. Petersburg Department of Steklov Mathematical Institute.

Author information

Authors and Affiliations

  1. Middle East Technical University, Ankara, Turkey

    S. Finashin

  2. Université de Strasbourg et IRMA (CNRS), Strasbourg, France

    V. Kharlamov

Authors
  1. S. Finashin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. V. Kharlamov
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to V. Kharlamov.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Finashin, S., Kharlamov, V. On wall-crossing invariance of certain sums of Welschinger numbers. Sel. Math. New Ser. 29, 41 (2023). https://doi.org/10.1007/s00029-023-00838-x

Download citation

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s00029-023-00838-x

Keywords

Mathematics Subject Classification

Search

Navigation