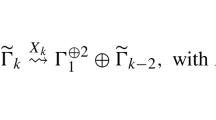Abstract
We provide necessary and sufficient conditions for the matrix equation \(X^\top A X=B\) to be consistent when B is a symmetric matrix, for all matrices A with a few exceptions. The matrices A, B, and X (unknown) are matrices with complex entries. We first see that we can restrict ourselves to the case where A and B are given in canonical form for congruence and, then, we address the equation with A and B in such form. The characterization strongly depends on the canonical form for congruence of A. The problem we solve is equivalent to: given a complex bilinear form (represented by A) find the maximum dimension of a subspace such that the restriction of the bilinear form to this subspace is a symmetric non-degenerate bilinear form.
Similar content being viewed by others
References
Benner, P., Palitta, D.: On the solution of the non-symmetric \(T\)-Riccati equation. Preprint. https://arxiv.org/pdf/2003.03693.pdf
Buckhiester, P.G.: Rank \(r\) solutions to the matrix equation \(XAX^t=C\), \(A\) alternate, over GF(\(2^y\)). Trans. Am. Math. Soc. 189, 201–209 (1974)
Buckhiester, P.G.: Rank \(r\) solutions to the matrix equation \(XAX^t=C\), \(A\) non-alternate, \(C\) alternate, over GF(\(2^y\)). Can. J. Math. 26, 78–90 (1974)
Buckhiester, P.G.: The number of solutions to the matrix equation \(XAX^{\prime }= C\), \(A\) and \(C\) nonalternate and of full rank, over GF(\(2^y\)). Math. Nachr. 63, 37–41 (1974)
Carlitz, L.: Representations by skew forms in a finite field. Arch. Math. 5, 19–31 (1954)
De Terán, F., Dopico, F.M.: The solution of the equation \(XA + AX^T = 0\) and its application to the theory of orbits. Linear Algebra Appl. 434, 44–67 (2011)
De Terán, F., Dopico, F.M.: Consistency and efficient solution for the Sylvester equation for \(*\)-congruence: \(AX+X^*B=C\). Electron. J. Linear Algebra 22, 849–863 (2011)
De Terán, F., Dopico, F.M., Guillery, N., Montealegre, D., Reyes, N.Z.: The solution of the equation \(AX+X^\star B=0\). Linear Algebra Appl. 438, 2817–2860 (2010)
De Terán, F., Iannazzo, B.: Uniqueness of solution of a generalized \(*\)-Sylvester matrix equation. Linear Algebra Appl. 493, 323–335 (2016)
De Terán, F., Iannazo, B., Poloni, F., Robol, L.: Solvability and uniqueness criteria for generalized Sylvester-type equations. Linear Algebra Appl. 542, 501–521 (2018)
De Terán, F., Iannazo, B., Poloni, F., Robol, L.: Nonsingular systems of generalized Sylvester equations: an algorithmic approach. Numer. Linear Algebra Appl. 26, e2261 (2019)
Ding, J., Rhee, N.H.: Spectral solutions of the Yang–Baxter matrix equation. J. Math. Anal. Appl. 402, 567–573 (2013)
Estatico, C., Di Benedetto, F.: Shift-invariant approximations of structured shift-variant blurring matrices. Numer. Algorithm 62, 615–635 (2013)
Fulton, J.D.: Generalized inverses of matrices over fields of characteristic two. Linear Algebra Appl. 28, 69–76 (1979)
Hodges, J.H.: A skew matrix equation over a finite field. Math. Nachr. 17, 49–55 (1966)
Horn, R.A., Johnson, C.R.: Matrix Analysis, 2nd edn. Cambridge University Press, New York (2013)
Horn, R.A., Sergeichuk, V.V.: Canonical forms for complex matrix congruence and \(*\)-congruence. Linear Algebra Appl. 416, 1010–1032 (2006)
Kressner, D., Liu, X.: Structured canonical forms for products of (skew-) symmetric matrices and the matrix equation \(XAX=B\). Electron. J. Linear Algebra 26, 215–230 (2013)
Turnbull, H.W., Aitken, A.C.: An Introduction to the Theory of Canonical Matrices. Dover, New York (1961). (First published by: Blackie & Son Limited, (1932))
Wei, H., Zhang, Y.: The number of solutions to the alternate matrix equation over a finite field and a \(q\)-identity. J. Stat. Plan. Inference 94, 349–358 (2001)
Acknowledgements
We thank an anonymous referee for several comments that allowed us to improve the original version. This work has been partially supported by the Ministerio de Economía y Competitividad of Spain through Grant MTM2015-65798-P (Secretaría de Estado de Investigación, Desarrollo e Innovación) (F. De Terán), and by the Ministerio de Ciencia, Innovación y Universidades of Spain through Grant MTM2017–90682–REDT.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Borobia, A., Canogar, R. & De Terán, F. On the Consistency of the Matrix Equation \(X^\top A X=B\) when B is Symmetric. Mediterr. J. Math. 18, 40 (2021). https://doi.org/10.1007/s00009-020-01656-7
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00009-020-01656-7
Keywords
- Matrix equation
- transpose
- congruence
- \(\top \)-Riccati equation
- canonical form for congruence
- symmetric matrix
- bilinear form