Skip to main content
SpringerLink
Log in

Attraction Region for the Classical Lotka−Volterra Predator−Prey model Caused by impulsive Effects

  • Published:
Qualitative Theory of Dynamical Systems Aims and scope Submit manuscript

Abstract

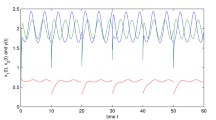
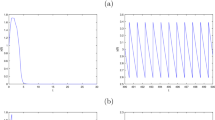
Discontinuous phenomena appear in various research fields. Impulsive differential equations are often used to model such discontinuous dynamics. This study deals with the Lotka–Volterra predator–prey model dominated by impulsive effects. In the modeling, impulses are added considering the ratios of the interior equilibrium to the current populations of the prey and predator. In this case, there is no restriction of the time interval between the impulse and the next impulse being the same. By focusing on the time interval between impulses adjacent to one another as well as the impulsive effect amount, a simple sufficient condition for the interior equilibrium to become globally asymptotically stable and sufficient conditions that are useful for estimating an attraction region are provided. Our results reveal that impulse control for the Lotka–Volterra predator–prey model can reduce the variation in the population of the prey and predator and enable the stable coexistence of both.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Data availability

All data analyzed during this study are included in this article.

References

  1. Bainov, D.D., Simeonov, P.S.: Systems with Impulse Effect: Stability Theory and Applications Ellis. Horwood Ser Mathematics and its Applications. Ellis Horwood Ltd., Chichester (1989)

    Google Scholar 

  2. Bainov, D.D., Simeonov, P.S.: Impulsive Differential Equations: Periodic Solutions and Applications. Pitman Monographs and Surveys in Pure and Applied Mathematics, vol. 66. Longman Scientific & Technical, Harlow (1993)

    Google Scholar 

  3. Bainov, D.D., Simeonov, P.S.: Impulsive Differential Equations, Asymptotic Properties of the Solutions. Series on Advances in Mathematics for Applied Sciences 28. World Scientific Publishing CoPte. Ltd., Singapore (1995)

    Book  Google Scholar 

  4. Barclay, H.J.: Models for pest control using predator release, habitat management and pesticide release in combination. J. Appl. Ecol. 19, 337–348 (1982)

    Article  Google Scholar 

  5. Brauer, F., Nohel, J.A.: The Qualitative Theory of Ordinary Differential Equations. Dover, New York (1989)

    MATH  Google Scholar 

  6. Caltagirone, L.E., Doutt, R.L.: The history of the vedalia beetle importation to California and its impact on the development of biological control. Ann. Rev. Entomol. 34, 1–16 (1989)

    Article  Google Scholar 

  7. Coppel, W.A.: Stability and Asymptotic Behavior of Differential Equations. Heath, Boston (1965)

    MATH  Google Scholar 

  8. Croft, B.A.: Arthropod Biological Control Agents and Pesticides. John Wiley & Sons Inc., New York (1990)

    Google Scholar 

  9. DeBach, P., Rosen, D.: Biological Control by Natural Enemies, 2nd edn. Cambridge University Press, Cambridge (1991)

    Google Scholar 

  10. Guo, H.-J., Song, X.: An impulsive predator-prey system with modified Leslie-Gower and Holling type II schemes. Chaos Solitons Fractals 36, 1320–1331 (2008)

    Article  MathSciNet  Google Scholar 

  11. Israel, G., Gasca, A.M.: The Biology Numbers: The Correspondence of Vito Volterra on Mathematical Biology. Science Networks Historical Studies, vol. 26. Birkhäuser Verlag, Basel (2002)

    Book  Google Scholar 

  12. Karsai, J.: Asymptotic behavior and its visualization of the solutions of intermittently and impulsively damped nonlinear oscillator equations Differential equations and computational simulations II. Appl. Math. Comput. 89, 161–172 (1998)

    MathSciNet  MATH  Google Scholar 

  13. Kellogg, R.L., Nehring, R., Grube, A., et al.: Environmental indicators of pesticide leaching and runoff from farm fields. In: Ball, V.E., Norton, G.W. (eds.) Agricultural Productivity Measurement and Sources of Growth, pp. 213–256. Kluwer Academic Publishers, Boston (2002)

    Chapter  Google Scholar 

  14. van Lenteren, J.C., Woets, J.: Biological and integrated pest control in greenhouses. Ann. Rev. Entomol. 33, 239–250 (1988)

    Article  Google Scholar 

  15. Liu, B., Teng, Z., Chen, L.-S.: Analysis of a predator-prey model with Holling II functional response concerning impulsive control strategy. J. Comput. Appl. Math. 193, 347–362 (2006)

    Article  MathSciNet  Google Scholar 

  16. Liu, B., Zhang, Y., Chen, L.-S.: The dynamical behaviors of a Lotka-Volterra predator-prey model concerning integrated pest management. Nonlinear Anal. Real World Appl. 6, 227–243 (2005)

    Article  MathSciNet  Google Scholar 

  17. Liu, J., Hu, J., Yuen, P.: Extinction and permanence of the predator-prey system with general functional response and impulsive control. Appl. Math. Model. 88, 55–67 (2020)

    Article  MathSciNet  Google Scholar 

  18. Lotka, A.J.: Undamped oscillations derived from the laws of mass action. J. Amer. Chem. Soc. 42, 1595–1599 (1920)

    Article  Google Scholar 

  19. Michel, A.N., Hou, L., Liu, D.: Stability Dynamical Systems: Continuous, Discontinuous, and Discrete Systems. Birkhäuser, Boston, Basel, Berlin (2008)

    MATH  Google Scholar 

  20. Pei, Y.-Z., Liu, S.-Y., Li, C.-G.: Complex dynamics of an impulsive control system in which predator species share a common prey. J. Nonlinear Sci. 19, 249–266 (2009)

    Article  MathSciNet  Google Scholar 

  21. Rouche, N., Habets, P., Laloy, M.: Stability Theory by Liapunov’s Direct Method. Applied Mathematical Sciences, vol. 22. Springer, Berlin (1977)

    Book  Google Scholar 

  22. Sugie, J.: Uniqueness of limit cycles in a predator-prey system with Holling-type functional response. Quart. Appl. Math. 58, 577–590 (2000)

    Article  MathSciNet  Google Scholar 

  23. Sugie, J., Katayama, M.: Global asymptotic stability of a predator-prey system of Holling type. Nonlinear Anal. 38, 105–121 (1999)

    Article  MathSciNet  Google Scholar 

  24. Sugie, J., Kohno, R., Miyazaki, R.: On a predator-prey system of Holling type. Proc. Amer. Math. Soc. 125, 2041–2050 (1997)

    Article  MathSciNet  Google Scholar 

  25. Sugie, J., Miyamoto, K., Morino, K.: Absence of limit cycles of a predator-prey system with a sigmoid functional response. Appl. Math. Lett. 9, 85–90 (1996)

    Article  MathSciNet  Google Scholar 

  26. Volterra, V.: Leçons sur la Théorie Mathématique de la Lutte pour la Vie. Gauthier-Villars, Paris (1931)

    MATH  Google Scholar 

  27. Wang, X.-Q., Wang, W.-M., Lin, X.-L.: Chaotic behavior of a Watt-type predator-prey system with impulsive control strategy. Chaos Solitons Fractals 37, 706–718 (2008)

    Article  MathSciNet  Google Scholar 

  28. Zhang, S., Dong, L.-Z., Chen, L.-S.: The study of predator-prey system with defensive ability of prey and impulsive perturbations on the predator. Chaos Solitons Fractals 23, 631–643 (2005)

    Article  MathSciNet  Google Scholar 

  29. Yoshizawa, T.: Stability Theory by Liapunov’s Second Method. Math. Soc. Japan, Tokyo (1966)

Download references

Acknowledgements

We would like to thank Editage (www.editage.com ) for their assistance with English language editing.

Funding

The first author’s work was supported in part by Japan Society for the Promotion of Science KAKENHI Grant-in-Aid for Scientific Research (C) [grant number JP20K03701].

Author information

Authors and Affiliations

  1. Department of Mathematics, Shimane University, 1060 Nishikawatsu-cho, Matsue, Shimane, 690-8504, Japan

    Jitsuro Sugie & Yoshiki Ishihara

Authors
  1. Jitsuro Sugie
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yoshiki Ishihara
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jitsuro Sugie.

Ethics declarations

Conflicts of interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Sugie, J., Ishihara, Y. Attraction Region for the Classical Lotka−Volterra Predator−Prey model Caused by impulsive Effects. Qual. Theory Dyn. Syst. 20, 46 (2021). https://doi.org/10.1007/s12346-021-00482-w

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s12346-021-00482-w

Keywords

Mathematics Subject Classification

Search

Navigation