Abstract
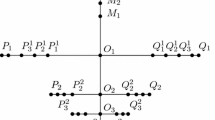
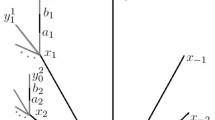
Let (X, d) be a compact metric space and f be a continuous map from X to X. Denote by R(f), \({ SA}(f)\) and \(\Gamma (f)\) the set of recurrent points, the set of special \(\alpha \)-limit points and the set of \(\gamma \)-limit points of f, respectively. It is well known that for an interval map f, the following three statements hold: (1) \(R(f)\subset { SA}(f)\cap \Gamma (f)\); (2) \({ SA}(f)= \Gamma (f)\); (3) \({ SA}(f)\cup \Gamma (f)\subset \overline{R(f)}\). The aim of this paper is to show that the above statement (1) holds for maps of dendrites with the number of endpoints being \(\aleph _{\mathbf{0}}\) (the cardinal number of the set of positive integers) and the above statements (2) and (3) do not hold for maps of dendrites with the number of endpoints being \(\aleph _{\mathbf{0}}\). Besides, we also study unilateral \(\gamma \)-limit points for maps of dendrites with the number of branch points being finite.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
Change history
24 July 2017
An erratum to this article has been published.
References
Acosta, G., Eslami, P.: On open maps between dendrites. Houst. J. Math. 33, 753–770 (2007)
Baldwin, S.: Continuous itinerary functions and dendrite maps. Topol. Appl. 154, 2889–2938 (2007)
Balibrea, F., Hric, R., Snoha, L.: Minimal sets on graphs and dendrites. Int. J. Bifurc. Chaos 13, 1721–1725 (2003)
Balibrea, F., Downarowicz, T., Hric, R., Snoha, L., Špitalský, V.: Almost totally disconnected minimal systems. Ergod. Theory Dyn. Syst. 29, 737–766 (2009)
Beardon, A.F.: Iteration of Rational Functions. Springer, New York (1991)
Chu, H., Xiong, J.: A counter example in dynamical systems of the interval. Proc. Am. Math. Soc. 97, 361–366 (1986)
Efremova, L.S., Makhrova, E.N.: The dynamics of monotone maps of dendrites. Sb. Math. 192, 807–821 (2001)
Efremova, L.S., Makhrova, E.N.: On the center of continuous maps of dendrites. J. Differ. Equ. Appl. 9, 381–392 (2003)
Hero, M.: Special \(\alpha \)-limit points for maps of the interval. Proc. Am. Math. Soc. 116, 1015–1022 (1992)
Kato, H.: A note on periodic points and recurrent points of maps of dendrites. Bull. Aust. Math. Soc. 51, 459–461 (1995)
Mai, J., Shi, E.: \(\overline{R} =\overline{P}\) for maps of dendrites \(X\) with Card(End(\(X\))) \(< c\). Int. J. Bifurc. Chaos 19, 1391–1396 (2009)
Nadler Jr., S.B.: Continuum Theory: An Introduction. Marcel Dekker Inc., New York (1992)
Naghmouchi, I.: Dynamical properties of monotone dendrite maps. Topol. Appl. 159, 144–149 (2012)
Naghmouchi, I.: Pointwise-recurrent dendrite maps. Ergod. Theory Dyn. Syst. 33, 1115–1123 (2013)
Peitgen, H.O., Richter, P.H.: The Beauty of Fractals. Springer, Berlin (1986)
Sun, T.: The set of unilateral \(\gamma \)-limit points and the topological entropy of a tree map (Chinese). Adv. Math. 33, 57–66 (2004)
Sun, T., Xi, H.: The centre and the depth of the centre for continuous maps on dendrites with finite branch points. Qual. Theory Dyn. Syst. (2016). doi:10.1007/s12346-016-0204-1
Sun, T., Xi, H., Chen, Z., Zhang, Y.: The attracting centre and the topological entropy of a graph map (Chinese). Adv. Math. 33, 540–546 (2004)
Sun, T., Su, G., Liang, H., He, Q.: Topological entropy and special \(\alpha \)-limit points of graph maps. Discret. Dyn. Nat. Soc. 2011, 1–7 (2011)
Sun, T., Xi, H., Liang, H.: Special \(\alpha \)-limit points and unilateral \(\gamma \)-limit points for graph maps. Sci. China Math. 54, 2013–2018 (2011)
Sun, T., He, Q., Xi, H.: Intra-orbit separation of dense orbits of dendrite maps. Chaos Solitons Fractals 57, 89–92 (2013)
Sun, T., Xi, H., He, Q.: Non-wandering sets of dendrite maps. Acta Math. Sin. Engl. Ser. (2016). doi:10.1007/s10114-016-5578-0
Xiong, J.: The attracting centre of a continuous self-map of the interval. Ergod. Theory Dyn. Syst. 8, 205–213 (1988)
Author information
Authors and Affiliations
Corresponding author
Additional information
An erratum to this article is available at https://doi.org/10.1007/s12346-017-0252-1.
Rights and permissions
About this article
Cite this article
Sun, T., Tang, Y., Su, G. et al. Special \(\alpha \)-Limit Points and \(\gamma \)-Limit Points of a Dendrite Map. Qual. Theory Dyn. Syst. 17, 245–257 (2018). https://doi.org/10.1007/s12346-017-0225-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s12346-017-0225-4