Skip to main content
SpringerLink
Log in

The dimension spectrum of conformal graph directed Markov systems

  • Published:
Selecta Mathematica Aims and scope Submit manuscript

Abstract

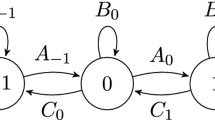
In this paper we study the dimension spectrum of general conformal graph directed Markov systems modeled by countable state symbolic subshifts of finite type. We perform a comprehensive study of the dimension spectrum addressing questions regarding its size and topological structure. As a corollary we prove that the dimension spectrum of infinite conformal iterated function systems is compact and perfect. On the way we revisit the role of the parameter \(\theta \) in graph directed Markov systems and we show that new phenomena arise. We also establish topological pressure estimates for subsystems in the abstract setting of symbolic dynamics with countable alphabets. These estimates play a crucial role in our proofs regarding the dimension spectrum, and they allow us to study Hausdorff dimension asymptotics for subsystems. Finally, we narrow our focus to the dimension spectrum of conformal iterated function systems and we prove, among other things, that the iterated function system resulting from the complex continued fractions algorithm has full dimension spectrum. As a result, we provide a positive answer to the Texan conjecture for complex continued fractions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

References

  1. Atnip, J.: Non-autonomous conformal graph directed Markov systems. arxiv:1706.09978

  2. Bonfiglioli, A., Lanconelli, E., Uguzzoni, F.: Stratified Lie Groups and Potential Theory for Their Sub-Laplacians, Springer Monographs in Mathematics. Springer, Berlin (2007)

    MATH  Google Scholar 

  3. Bowen, R.: Hausdorff dimension of quasicircles. Inst. Hautes Études Sci. Publ. Math. 50, 11–25 (1979)

    Article  MathSciNet  Google Scholar 

  4. Capogna, L., Danielli, D., Pauls, S., Tyson, J.: An Introduction to the Heisenberg Group and the Sub-Riemannian Isoperimetric Problem, Progress in Mathematics, vol. 259. Birkhäuser Verlag, Basel (2007)

    MATH  Google Scholar 

  5. Chousionis, V., Tyson, J. T., Urbański, M.: Conformal graph directed Markov systems on Carnot groups. Mem. AMS

  6. Cusick, T.W.: Hausdorff dimension of sets of continued fractions. Q. J. Math. Oxf. Ser. (2) 41(163), 277–286 (1990)

    Article  MathSciNet  Google Scholar 

  7. Dajani, K., Hensley, D., Kraaikamp, C., Masarotto, V.: Arithmetic and ergodic properties of ‘flipped’ continued fraction algorithms. Acta Arith. 153(1), 51–79 (2012)

    Article  MathSciNet  Google Scholar 

  8. Edgar, G.A., Mauldin, D.: Multifractal decompositions of digraph recursive fractals. Proc. Lond. Math. Soc. 3(65), 604–628 (1992)

    Article  MathSciNet  Google Scholar 

  9. Falk, R.S., Nussbaum, R.D.: A new approach to numerical computation of Hausdorff dimension of iterated function systems: applications to complex continued fractions. Integral Equ. Oper. Theory 90(5), 90–61 (2018)

    Article  MathSciNet  Google Scholar 

  10. Falk, R.S., Nussbaum, R.D.: \(C^m\) eigenfunctions of Perron–Frobenius operators and a new approach to numerical computation of Hausdorff dimension: applications in R1. J. Fractal Geom. 5(3), 279–337 (2018)

    Article  MathSciNet  Google Scholar 

  11. Good, I.J.: The fractional dimensional theory of continued fractions. Proc. Camb. Philos. Soc. 37, 199–228 (1941)

    Article  MathSciNet  Google Scholar 

  12. Ghenciu, A., Munday, S., Roy, M.: The Hausdorff dimension spectrum of conformal graph directed Markov systems and applications to nearest integer continued fractions. J. Number Theory 175, 223–249 (2017)

    Article  MathSciNet  Google Scholar 

  13. Heinemann, S., Urbański, M.: Hausdorff dimension estimates for infinite conformal iterated function systems. Nonlinearity 15, 727–734 (2002)

    Article  MathSciNet  Google Scholar 

  14. Hensley, D.: Continued fraction Cantor sets, Hausdorff dimension, and functional analysis. J. Number Theory 40(3), 336–358 (1992)

    Article  MathSciNet  Google Scholar 

  15. Hensley, D.: A polynomial time algorithm for the Hausdorff dimension of continued fraction Cantor sets. J. Number Theory 58(1), 9–45 (1996)

    Article  MathSciNet  Google Scholar 

  16. Hensley, D.: Continued Fractions. World Scientific Publishing Co Pte. Ltd., Hackensack (2006)

    Book  Google Scholar 

  17. Hensley, D.: Continued fractions, Cantor sets, Hausdorff dimension, and transfer operators and their analytic extension. Discrete Contin. Dyn. Syst. 32(7), 2417–2436 (2012)

    Article  MathSciNet  Google Scholar 

  18. Hurwitz, A.: Über die Entwicklung complexer Grössen in Kettenbrüche. Acta Math. XI, 187–200 (1888)

    MATH  Google Scholar 

  19. Hurwitz, J.: Über eine besondere Art der Kettenbruch-Entwicklung complexer Grössen. Dissertation, University of Halle (1895)

  20. Jenkinson, O.: On the density of Hausdorff dimensions of bounded type continued fraction sets: the Texan conjecture. Stoch. Dyn. 4(1), 63–76 (2004)

    Article  MathSciNet  Google Scholar 

  21. Jenkinson, O., Pollicott, M.: Computing the dimension of dynamically defined sets: E2 and bounded continued fractions. Ergod. Theory Dyn. Syst. 21, 1429–1445 (2001)

    MATH  Google Scholar 

  22. Jenkinson, O., Pollicott, M.: Rigorous effective bounds on the Hausdorff dimension of continued fraction Cantor sets: a hundred decimal digits for the dimension of E2, Adv. Math, to appear

  23. Kesseböhmer, M., Zhu, S.: Dimension sets for infinite IFSs: the Texan conjecture. J. Number Theory 1(116), 230–246 (2006)

    Article  MathSciNet  Google Scholar 

  24. Kesseböhmer, M., Kombrink, S.: Minkowski content and fractal Euler characteristic for conformal graph directed systems. J. Fractal Geom. 2(2), 171–227 (2015)

    Article  MathSciNet  Google Scholar 

  25. Kesseböhmer, M., Kombrink, S.: Minkowski measurability of infinite conformal graph directed systems and application to Apollonian packings. arXiv:1702.02854

  26. Mauldin, D., Urbański, M.: Dimensions and measures in infinite iterated function systems. Proc. Lond. Math. Soc. (3) 73(1), 105–154 (1996)

    Article  MathSciNet  Google Scholar 

  27. Mauldin, D., Urbański, M.: Conformal iterated function systems with applications to the geometry of conformal iterated function systems. Trans. Am. Math. Soc. 351, 4995–5025 (1999)

    Article  Google Scholar 

  28. Mauldin, D., Urbański, M.: Graph Directed Markov Systems: Geometry and Dynamics of Limit Sets, Cambridge Tracts in Mathematics, vol. 148. Cambridge University Press, Cambridge (2003)

    Book  Google Scholar 

  29. Mauldin, D., Williams, S.C.: Hausdorff dimension in graph directed constructions. Trans. Am. Math. Soc. 309, 811–829 (1988)

    Article  MathSciNet  Google Scholar 

  30. McMullen, C.T.: Hausdorff dimension and conformal dynamics. Computation of dimension, III. Am. J. Math. 120(4), 691–721 (1998)

    Article  Google Scholar 

  31. Pollicott, M., Urbański, M.: Asymptotic Counting in Conformal Dynamical Systems. arXiv:1704.06896

  32. Priyadarshi, A.: Lower bound on the Hausdorff dimension of a set of complex continued fractions. J. Math. Anal. Appl. 449(1), 91–95 (2017)

    Article  MathSciNet  Google Scholar 

  33. Roy, M.: A new variation of Bowen’s formula for graph directed Markov systems. Discrete Contin. Dyn. Syst. 32(7), 2533–2551 (2012)

    Article  MathSciNet  Google Scholar 

  34. Rump, S.M.: INTLAB—INTerval LABoratory, Developments in Reliable Computing, pp. 77–104 (1999)

    Chapter  Google Scholar 

  35. Rump, S.M.: Verification methods: rigorous results using floating-point arithmetic. Acta Numer. 19, 287–449 (2010)

    Article  MathSciNet  Google Scholar 

  36. Schmidt, A.: Diophantine approximation of complex numbers. Acta Math. 134, 1–85 (1975)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, University of Connecticut, Storrs, USA

    Vasileios Chousionis & Dmitriy Leykekhman

  2. Department of Mathematics, University of North Texas, Denton, USA

    Mariusz Urbański

Authors
  1. Vasileios Chousionis
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Dmitriy Leykekhman
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Mariusz Urbański
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Vasileios Chousionis.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Vasileios Chousionis was supported in part by the Simons Foundation Collaboration Grant No. 521845. Dmitriy Leykekhman was supported in part by the NSF Grant No. DMS-1522555. Mariusz Urbański was supported in part by the NSF Grant No. DMS-1361677.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Chousionis, V., Leykekhman, D. & Urbański, M. The dimension spectrum of conformal graph directed Markov systems. Sel. Math. New Ser. 25, 40 (2019). https://doi.org/10.1007/s00029-019-0487-6

Download citation

  • Published:

  • DOI: https://doi.org/10.1007/s00029-019-0487-6

Mathematics Subject Classification

Search

Navigation