Reliability of Birolini’s duplex system sustained by a cold standby unit and subjected to a priority rule

Abstract

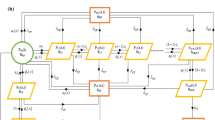
We analyse the survival time of Birolini’s duplex system sustained by a cold standby unit subjected to a priority rule. We employ a stochastic process endowed with time-dependent transition measures satisfying coupled partial differential equations leading to the Laplace transform of the survival function. As an example, we consider Coxian distributions for failure and repair. Some graphs are displayed together with a security interval corresponding to a security level of 90 %.

Appendices

Appendix A

For direct reference, we propose to state some particular properties of sectionally holomorphic functions and their ramifications for the solution of some boundary value problems on the real line. See Gakhov (1996, pp. 1–40), Roos (1996, pp. 118–242) for proofs and details. Let $\varphi (\tau )$ be a function satisfying the Hölder (Lipschitz) condition on R and at infinity. In addition, let

$$\begin{aligned} \mathcal {L}^+(u)&:= \lim _{\begin{array}{c} \omega \rightarrow u\\ \omega \in \mathbf {C}^+ \end{array}}\,\,\frac{1}{2\pi i}\,\int _{\Gamma }\,\varphi (\tau )\frac{\mathrm{d}\tau }{\tau -\omega },\,\,u\in \mathbf {R},\\ \mathcal {L}^-(u)&:= \lim _{\begin{array}{c} \omega \rightarrow u\\ \omega \in \mathbf {C}^- \end{array}}\,\,\frac{1}{2\pi i}\,\int _{\Gamma }\,\varphi (\tau )\frac{\mathrm{d}\tau }{\tau -\omega },\,\,u\in \mathbf {R}. \end{aligned}$$

We have

$$\begin{aligned} \mathcal {L}^+(u)&= \frac{1}{2}\varphi (u)+\frac{1}{2\pi i}\,\int _{\Gamma }\,\varphi (\tau )\frac{\mathrm{d}\tau }{\tau -u}.\end{aligned}$$

(9.1)

$$\begin{aligned} \mathcal {L}^-(u)&= -\frac{1}{2}\varphi (u)+\frac{1}{2\pi i}\,\int _{\Gamma }\,\varphi (\tau )\frac{\mathrm{d}\tau }{\tau -u}, \end{aligned}$$

(9.2)

Hence, for $u\in \mathbf {R}$

$$\begin{aligned} \mathcal {L}^+(u)-\mathcal {L}^-(u)&= \varphi (u),\end{aligned}$$

(9.3)

$$\begin{aligned} \frac{\mathcal {L}^+(u)+\mathcal {L}^-(u)}{2}&= \frac{1}{2\pi i}\,\int _\Gamma \,\,\varphi (\tau )\,\frac{\mathrm{d}\tau }{\tau -u}. \end{aligned}$$

(9.4)

The relations (9.1)–(9.4) are called the Sokhotski–Plemelj formulas on the real line. The functions $\mathcal {L}^+(u),\,\mathcal {L}^{-}(u)$ are continuous on R and infinity. The function $\varphi (\tau )$ has a unique decomposition and the resulting boundary value Eq. (9.3) has a unique regular solution

$$\begin{aligned} \frac{1}{2\pi i}\,\,\int _\Gamma \,\varphi (\tau )\frac{\mathrm{d}\tau }{\tau -\omega }. \end{aligned}$$

valid for all $\omega \in \mathbf {C}$ and the Cauchy-type integral generates a regular sectionally holomorphic function in C cut along the real line. Furthermore,

$$\begin{aligned} \mathcal {L}^+(\omega )&= \int _\Gamma \,\,\varphi (\tau )\frac{\mathrm{d}\tau }{\tau -\omega },\,\,\omega \in \mathbf {C}^+,\\ \mathcal {L}^-(\omega )&= \int _\Gamma \,\,\varphi (\tau )\frac{\mathrm{d}\tau }{\tau -\omega },\,\,\omega \in \mathbf {C}^-. \end{aligned}$$

Appendix B

To present a comprehensive derivation of the Eqs. (5.1)–(5.5), we first proceed to the Laplace transformation of Eq. (4.7). i.e.

$$\begin{aligned} \int ^\infty _0 \mathrm{e}^{-zt}\left( \lambda _\mathrm{w}+\frac{\partial }{\partial t}-\frac{\partial }{\partial x}\right) p_A^*(t,x)=p_B^*(z,x,0)+p_{B_\mathrm{w}}^*(z,x,0),\,\,\text {Re}\,z>0. \end{aligned}$$

Applying the product rule (partial integration) yields

$$\begin{aligned} \int ^\infty _0 \mathrm{e}^{-zt}\frac{\partial p_A(t,x)}{\partial t} \,\mathrm{d}t=\mathrm{e}^{-zt}p_A(t,x) \biggr |^\infty _0+z\int ^\infty _0 \mathrm{e}^{-zt}p_A(t,x) \,\mathrm{d}t. \end{aligned}$$

Note that the initial condition $N_0=A, x_0=f$ implies that

$$\begin{aligned} \mathrm{e}^{-zt}p_A(t,x) \biggr |^\infty _0=-p_A(0,x)=-\frac{\mathrm{d}F}{\mathrm{d}x}. \end{aligned}$$

Hence,

$$\begin{aligned} (\lambda _\mathrm{w}\!+\!z)\int ^\infty _0 \mathrm{e}^{-zt}p_A(t,x) \,\mathrm{d}t \!+\! \int ^\infty _0 \frac{\partial p_A(t,x)}{\partial t} \mathrm{d}t \!=\! p_B^*(z,x,0)\!+\!p_{B_\mathrm{w}}^*(z,x,0)\!+\!\frac{\mathrm{d}F}{\mathrm{d}x}.\nonumber \\ \end{aligned}$$

(10.1)

Next, we transform Eq. (10.1). Changing the order of integration, justified by the Tonelli–Hobson test, e.g. Apostol (1978, page 415) and applying the product rule again, taking the property $p_A(\cdot ,\infty )=0$ into account, entails that

$$\begin{aligned}&(\lambda _\mathrm{w}+z+i\zeta ) \int ^\infty _0 \mathrm{e}^{-zt} \int ^\infty _0 \mathrm{e}^{-\zeta x} p_A(t,x)\,\mathrm{d}x\mathrm{d}t+p_A^*(z,0)\\&\quad =\int ^\infty _0 \mathrm{e}^{-\zeta x} p_B^*(z,x,0)\,\mathrm{d}x+\int ^\infty _0 \mathrm{e}^{-\zeta x} p_{B_\mathrm{w}}^*(z,x,0)\,\mathrm{d}x+\mathbf {E}\mathrm{e}^{i \zeta f}, \,\,\, \text {Im}\,\zeta >0. \end{aligned}$$

Finally, note that the definition and properties of the $\mathbf {E}$ operator imply that

$$\begin{aligned} \int ^\infty _0 \mathrm{e}^{-\zeta x} p_A(t,x)\,\mathrm{d}x=\mathbf {E}(\mathrm{e}^{-\zeta X_t} \mathbf {1}\{N_t=A\}). \end{aligned}$$

The Eqs. (5.2–5.5) are obtained in a similar way. For instance, observe that

$$\begin{aligned} \int ^\infty _0 \int ^\infty _0 \mathrm{e}^{-\zeta x} \mathrm{e}^{-\eta y} p_B(t,x,y)\,\mathrm{d}x\mathrm{d}y=\mathbf {E}(\mathrm{e}^{i\zeta X_t}\mathrm{e}^{i\eta Y_t} \mathbf {1}\{N_t=B\}). \end{aligned}$$

independent of the order of integration.