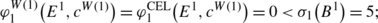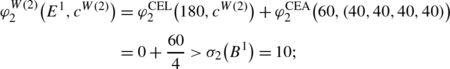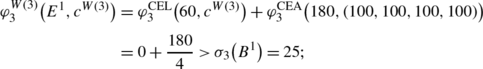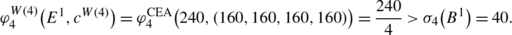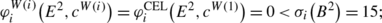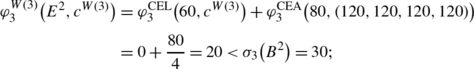The minimal overlap rule: restrictions on mergers for creditors’ consensus

Abstract

As it is known, there is no rule satisfying additivity in the complete domain of bankruptcy problems. This paper proposes a notion of partial additivity in this context, to be called μ-additivity. We find out that this property, together with two quite compelling axioms, equal treatment of equals and continuity, identify the minimal overlap rule, introduced by O’Neill (Math. Soc. Sci. 2:345–371, 1982).

Appendices

Appendix A: General remark

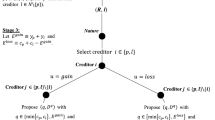
We note how to check if a creditor $i\in \mathcal{N}$ is privileged (Definition 6) when facing a problem B=(E,c). This reasoning will be used in Examples 2 and 3 as well as in Appendix B.

Let us introduce the constrained equal awards rule, which will be useful in the sequel. This rule is the function $\varphi^{\mathrm{CEA}} \colon \mathcal{B}\rightarrow \mathbb{R}_{+}^{n}$ that associates, to each problem $(E, c ) \in \mathcal{B}$ and each creditor $i \in \mathcal{N}$, the amount

where α is such that $\sum_{i\in \mathcal{N}}\min \{c_{i}, \alpha \} = E$.

We define, for each creditor $i\in \mathcal{N}$, the bankruptcy solution $\varphi^{W (i )}: \mathcal{B} \rightarrow \mathbb{R}_{+}^{n}$ that associates, to each $(E, c ) \in \mathcal{B}$ and each creditor $j \in \mathcal{N}$, the amount

where

This rule will be useful to determine the worst situation that agent i might face when distributing the estate. In what follows, we describe which situation will be understood as the worst one for agent i. Let us remember that we assume that creditors’ claims are increasingly ordered.

First, let us note that for each $(E, c ) \in \mathcal{B}$, and each $i \in \mathcal{N}$,

This follows from the way in which φ ^W(i) is described. Now, for each problem $(E, c ) \in \mathcal{B}$ and creditor i, let us denote

Finally, let us associate, to each $(E, c ) \in \mathcal{B}$ and $i \in \mathcal{N}$, vector c ^W(i) such that for each $j \in \mathcal{N}$,

This implies that

From the previous facts we have that, for each $(E, c ) \in \mathcal{B}$ and $i \in \mathcal{N}$, φ ^W(i)(E,c ^W(i)) will provide the smallest part of the estate for agent i among all the minimally fair rules and all the configurations of claims in C′(i). We call the pair (φ ^W(i),c ^W(i)) the worst situation for agent i, since it corresponds to the least favorable situation for such a creditor with regard to both the rest of agents’ claims demanding less than she does and the rule that prevails. Therefore, we can establish:

Remark 1

To prove that creditor i is privileged it will be enough to check that some of the conditions, (a) or (b), in Definition 6 is satisfied in the worst situation for such a creditor.

To illustrate the notion of privileged creditor we present, taking into account Remark 1, the partition of the set of creditors according to such a qualification for two different problems. In the first one, all the claims are lower than the estate, whereas in the second problem, there is some creditor who claims more than the debtor’s assets.

Example 2

Let B ¹=(E ¹,c ¹)=(240,(20,40,100,160)). By definition, the security levels vector is

and the claims vectors c ^W(i) are

Therefore,

• (1) creditor 1 is non-privileged since

  

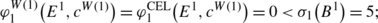
• (2) creditor 2 is privileged since

  

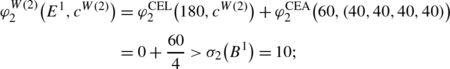
• (3) creditor 3 is also privileged since

  

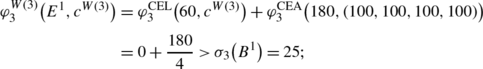
• (4) finally, creditor 4 is privileged since

  

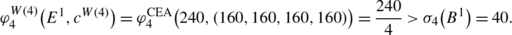
Example 3

Let B ²=(E ²,c ²)=(140,(60,60,120,180)). By definition, the security levels vector is

and the claims vectors c ^W(i) are

Therefore, we have that

• (1) creditors 1 and 2 are non-privileged since for i=1,2,

  

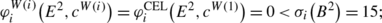
• (2) creditor 3 is also non-privileged since

  

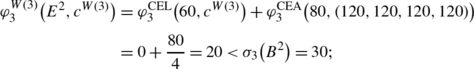
• (3) finally, creditor 4 is privileged since

  

Appendix B: Proof of Proposition 1

Firstly we show that Definition 6 implies conditions (a) and (b) of Proposition 1.

By Remark 1 we have that it is sufficient to check that the consequence holds for φ ^W(i)(E,c ^W(i)).

Let (E,c) be a problem, and $i \in \mathcal{N}$ be a creditor. If i is privileged in (E,c) then E>∑ _j>i (c _j −c _i ); otherwise, $\varphi^{W (i )}_{i} (E, c^{W (i )} ) = 0$ and neither (a) nor (b) in Definition 6 would be met. Thus, for each privileged agent i,

(4)

Let $i \in \mathcal{N}$ be a privileged creditor in $(E, c ) \in \mathcal{B}$. We have one of the two following cases.

Case 1

$\varphi^{W (i )}_{i} (E, c^{W (i )} ) > \sigma_{i} (E, c )$.

By Eq. (4), we have that

which implies that

Let us define

and let $S \subset \mathcal{N}$ be the subset of creditors, whose cardinality is denoted by s, such that k∈S if and only if k≠i and c _k ≥c _i , and let $\beta = \frac{1}{s}\min \{c_{i}, \alpha \}$. Now we define $\tau \in \mathbb{R}_{+}^{n}$ such that

Thus

is a problem in $\mathcal{B}$ and for all $j \in \mathcal{N}$, j≠i, $\min \{c_{i}^{\prime}, E^{\prime} \} > \min \{c_{j}^{\prime}, E^{\prime} \}$.

Case 2

$\varphi_{i}^{W (i )} (E, c^{W (i )} ) = \sigma_{i} (E, c )$, and σ _i (E,c)>σ _j (E,c) for all $j \in \mathcal{N}$, j≠i.

By Definition 5, the previous inequality is equivalent to $c_{i}^{E} > c_{j}^{E}$ for all $j \in \mathcal{N}$, j≠i. Therefore, for $\tau \in \mathbb{R}_{+}^{n}$ such that τ _i =0 for all $i \in \mathcal{N}$, conditions (a) and (b) of Proposition 1 are satisfied.

Secondly we show that conditions (a) and (b) of Proposition 1 imply Definition 6.

Let us suppose that there is $\tau \in \mathbb{R}_{+}^{n}$ such that

• (a) $B^{\prime} = (E^{\prime}, c^{\prime} ) = (E - \sum_{h = 1}^{n}\tau_{h}, c - \tau ) \in \mathcal{B}$, and

• (b) $\min \{c_{i}^{\prime}, E^{\prime} \} > \min \{c_{j}^{\prime}, E^{\prime} \}$ for all $j \in \mathcal{N}$, j≠i.

This implies that for all $j \in \mathcal{N}$, j≠i, either $E^{\prime} \geq c_{i}^{\prime} > c_{j}^{\prime}$ or $c_{i}^{\prime} > E^{\prime} > c_{j}^{\prime}$. Therefore, there is τ such that in the residual problem, (E′,c′), $c_{i}^{\prime}>c_{j}^{\prime}$ for all $j \in \mathcal{N}$. Let us look for the minimum partial reimbursement of debts, τ ^∗, for which

Let us consider the following two cases.

Case 1

c _i =c _n >c _n−1.

Then τ ^∗=0 and, by Remark 1, φ ^W(i)=φ ^CEA. If E>c _i ,

and condition (a) in Definition 6 is satisfied. Otherwise, i.e. if c _i >E>c _n−1,

which implies condition (b) in Definition 6.

Case 2

There is $j \in \mathcal{N}$, j≠i such that c _j ≥c _i .

Then it is possible to partially reimburse debts in such a way that: (i) no creditor has a new claim greater than creditor i does, and (ii) what is left of estate is greater than c _i . Otherwise conditions (a) and (b) of Proposition 1 would not be fulfilled. Therefore, the previous statement is equivalent to

where $\tau^{\ast} \in \mathbb{R}_{+}^{n}$ is such that $\tau_{h}^{\ast} = \max \{c_{h} - c_{i}, 0 \}$ for all $h \in \mathcal{N}$.

From the previous inequality,

Now, taking into account Remark 1,

which implies condition (a) in Definition 6.

Appendix C: Proof of Proposition 2

Let φ be a rule satisfying μ-additivity and continuity. Let (E,c) be a problem where $0 < E <c_{n} = \max_{i \in \mathcal{N}} \{c_{i} \}$; and let $S\subset \mathcal{N}$ be the subset of agents claiming zero. Let us consider the following two cases.

Case 1

E≤c _n−1.

By μ-additivity,

for all r∈ℕ such that

By continuity,

Case 2

E>c _n−1.

By μ-additivity,

for all r∈ℕ such that

Therefore, by continuity,

Appendix D: Proof of Theorem 1

Firstly, it is straightforward to verify that the minimal overlap rule satisfies equal treatment of equals, continuity and μ-additivity.

Now, let φ be a rule satisfying these axioms. Given a problem $(E, c ) \in \mathcal{B}$, let us consider the following two cases.

Case 1

E≤c _n .

By Proposition 2,

Let us denote $P^{1} = c_{1}^{E}$; $P^{i} = c_{i}^{E} - c_{i - 1}^{E}$ for 1<i≤n and $c^{P^{h}} = ( (0 )_{j < h}, (P^{h} )_{j \geq h} )$, for each $h \in \mathcal{N}$.

Now, let us consider the following two subcases.

Subcase (a)

$c^{E}_{n} = c^{E}_{n - 1}$.

μ-additivity implies that

By equal treatment of equals and Proposition 2,

i.e.

with c ₀=0.⁸ Thus, for each agent h,

Since, for any j>h we have that $c_{h}^{P^{j}}=0$,

Subcase (b)

$c^{E}_{n} \neq c^{E}_{n-1}$.

Let q(j) denote the cardinality of the set {i≤j:P ⁱ≠0}. μ-Additivity implies that

where r∈ℕ is such that

Now, by continuity,

By using the reasoning of the Subcase (a) above, we obtain that for each agent h,

Case 2

E>c _n .

In such a case, there is a unique t, 0≤t<c _n , such that

Let k be the unique agent such that c _k −t>0, and c _k−1−t≤0. Note that this implies that each agent j, with j≥k, is privileged in (E,c). Then, for each r∈ℕ such that

by μ-additivity,

(5)

by continuity,

Observe that the problem $( t, ( \min \{ c_{i},t \} )_{i\in \mathcal{N}} )$ was analyzed in Case 1 above. Therefore, for each agent h,

$$ \varphi_{h} \bigl( t, \bigl( \min \{ c_{i},t \} \bigr)_{i\in \mathcal{N}} \bigr) =\sum_{i=1}^{h} \frac{\min \{ c_{i},t \} -\min \{ c_{i-1},t \} }{n-i+1}. $$

(6)

Moreover, note that for agent h,

$$ \max \{ 0,c_{h}-t \} =\left \{ \begin{array}{l@{\quad}l} 0 & \mbox{if $h < k$,} \\ c_{h}-t & \mbox{\ if $h \geq k$.}\end{array} \right . $$

Since by construction,

$$ \sum_{i=1}^{n}\max \{ 0,c_{i}-t \} =E-t, $$

we conclude that for each agent h,

$$ \varphi_{h} \bigl( E-t, \bigl( \max \{ 0,c_{i}-t \} \bigr)_{i\in \mathcal{N}} \bigr) =\max \{ 0,c_{h}-t \} . $$

(7)

Finally, by combining Eqs. (5), (6), and (7), we have that, for each agent h,

$$ \varphi_{h} ( E,c ) =\sum_{i=1}^{h} \frac{\min \{ c_{i},t \} -\min \{ c_{i-1},t \} }{n-i+1}+\max \{ 0,c_{h}-t \} =\varphi_{h}^{\mathrm{MO}} ( E,c ) . $$