Skip to main content
SpringerLink
Log in

A combinatorial formula for affine Hall–Littlewood functions via a weighted Brion theorem

  • Published:
Selecta Mathematica Aims and scope Submit manuscript

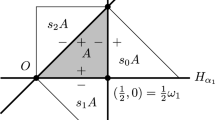
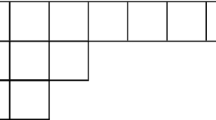
Abstract

We present a new combinatorial formula for Hall–Littlewood functions associated with the affine root system of type \({{\tilde{A}}}_{n-1}\), i.e., corresponding to the affine Lie algebra \({{\widehat{\mathfrak {sl}}}}_n\). Our formula has the form of a sum over the elements of a basis constructed by Feigin, Jimbo, Loktev, Miwa and Mukhin in the corresponding irreducible representation. Our formula can be viewed as a weighted sum of exponentials of integer points in a certain infinite-dimensional convex polyhedron. We derive a weighted version of Brion’s theorem and then apply it to our polyhedron to prove the formula.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3

Similar content being viewed by others

References

  1. Carter, R.: Lie Algebras of Finite and Affine Type. Cambridge University Press, Cambridge (2005)

    Book  MATH  Google Scholar 

  2. Viswanath, S.: Kostka–Foulkes polynomials for symmetrizable Kac-Moody algebras. Sém. Lothar. Combin. 58:Art, B58f (2008)

  3. Fishel, S., Grojnowski, I., Teleman, C.: The strong Macdonald conjecture and Hodge theory on the Loop Grassmannian. Ann. Math. 168(1), 175–220 (2008)

    Article  MathSciNet  Google Scholar 

  4. Cherednik, I.: A New Take on Spherical, Whittaker and Bessel Functions (2009). arXiv:0904.4324v2

  5. Macdonald, I.G.: Symmetric Functions and Hall Polynomials, Oxford Mathematical Monographs, 2nd edn. Oxford University Press, New York (1995)

    Google Scholar 

  6. Stoyanovsky, A.V., Feigin, B.L.: Functional models for representations of current algebras and semi-infinite Schubert cells. Funct. Anal. Its Appl. 28(1), 55–72 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  7. Feigin, B., Jimbo, M., Loktev, S., Miwa, T., Mukhin, E.: Bosonic formulas for (k, l)-admissible partitions. Ramanujan J. 7(4), 485–517 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  8. Feigin, B., Jimbo, M., Loktev, S., Miwa, T., Mukhin, E.: Addendum to ‘Bosonic formulas for (k, l)-admissible partitions’. Ramanujan J. 7(4), 519–530 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  9. Brion, M.: Points entiers dans les polyédres convexes. Ann. Sci. École Norm. Sup. 21(4), 653–663 (1988)

    MathSciNet  MATH  Google Scholar 

  10. Makhlin, I.: Weyl’s Formula as the Brion Theorem for Gelfand-Tsetlin Polytopes (2014). arXiv:1409.7996

  11. Makhlin, I.: Characters of Feigin-Stoyanovsky subspaces and Brion’s theorem. Funct. Anal. Its Appl. 49(1), 15–24 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  12. Jimbo, M.: Quantum R Matrix Related to the Generalized Toda System: An Algebraic Approach, Lecture Notes in Physics, vol. 246, pp. 335–361. Springer, Berlin (1986)

  13. Feigin, B., Finkelberg, M., Negut, A., Rybnikov, L.: Yangians and cohomology rings of Laumon spaces. Selecta Math. 17(3), 573–607 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  14. Tsymbaliuk, A.: Quantum affine Gelfand-Tsetlin bases and quantum toroidal algebra via K-theory of affine Laumon spaces. Selecta Math. 16(2), 173–200 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  15. Pukhlikov, A.V., Khovanskii, A.G.: Finitely additive measures of virtual polyhedra. Algebra i Analiz 4(2), 161–185 (1992)

    MathSciNet  MATH  Google Scholar 

  16. Barvinok, A.I.: Integer Points in Polyhedra. European Mathematical Society (EMS), Zürich (2008)

    Book  MATH  Google Scholar 

  17. Beck, M., Robins, S.: Computing the Continuous Discretely. Springer, New York (2009)

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. International Laboratory of Representation Theory and Mathematical Physics, National Research University Higher School of Economics, Myasnitskaya ulitsa 20, Moscow, Russia, 101000

    Boris Feigin & Igor Makhlin

  2. Landau Institute for Theoretical Physics, prospekt Akademika Semenova 1A, Chernogolovka, Russia, 142432

    Boris Feigin & Igor Makhlin

Authors
  1. Boris Feigin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Igor Makhlin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Igor Makhlin.

Additional information

Igor Makhlin: Supported in part by the Simons Foundation and the Moebius Contest Foundation for Young Scientists.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Feigin, B., Makhlin, I. A combinatorial formula for affine Hall–Littlewood functions via a weighted Brion theorem. Sel. Math. New Ser. 22, 1703–1747 (2016). https://doi.org/10.1007/s00029-016-0223-4

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00029-016-0223-4

Keywords

Search

Navigation