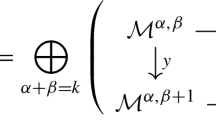Abstract
Let \(X\) be a smooth variety over an algebraically closed field of characteristic \(p > 0, Z\) a smooth divisor, and \(j: U=X {\setminus } Z \rightarrow X\) the natural inclusion. We introduce in an axiomatic way the notion of a \(V\)-filtration on unit \(F\)-crystals and prove such axioms determine a unique filtration. It is shown that if \(\mathcal M \) is a tame unit \(F\)-crystal on \(U\), then such a \(V\)-filtration along \(Z\) exists on \(j_*\mathcal M \). The degree zero component of the associated graded module is proven to be the (unipotent) nearby cycles functor of Grothendieck and Deligne under the Emerton–Kisin Riemann–Hilbert correspondence. A few applications to \(\mathbb A ^1\) and gluing are then discussed.
Similar content being viewed by others
References
Altman, A., Kleiman, S.L.: On the purity of the branch locus. Compositio Math. 23, 461–465 (1971)
Beilinson, A.A.: How to glue perverse sheaves. In: \(K\)-theory, Arithmetic and Geometry (Moscow, 1984–1986), vol. 1289 of Lecture Notes in Mathematics, pp. 42–51. Springer, Berlin (1987)
Deligne, P., Katz, N.: Groupes de monodromie en géométrie algébrique. I. In: Lecture Notes in Mathematics, vol. 340. Springer, Berlin. Séminaire de Géométrie Algébrique du Bois-Marie 1967–1969 (SGA 7 II) (1973)
Derksen, H., Weyman, J.: Quiver representations. Notices Am. Math. Soc. 52(2), 200–206 (2005)
Eisenbud, D.: Commutative Algebra, vol. 150 of Graduate Texts in Mathematics. Springer, New York. With a view toward algebraic geometry (1995)
Emerton, M., Kisin, M.: The Riemann–Hilbert correspondence for unit \(F\)-crystals. Astérisque, 93, vi+257 (2004)
Gabber, O.: Notes on some \(t\)-structures. In: Geometric Aspects of Dwork Theory, vols. I, II, pp. 711–734. Walter de Gruyter GmbH & Co. KG, Berlin (2004)
Grothendieck, A., Murre, J.P.: The tame fundamental group of a formal neighbourhood of a divisor with normal crossings on a scheme. In: Lecture Notes in Mathematics, vol. 208. Springer, Berlin (1971)
Grothendieck, A.: Revêtements étales et groupe fondamental. Fasc. I: Exposés 1 à 5, vol. 1960/61 of Séminaire de Géométrie Algébrique. Institut des Hautes Études Scientifiques, Paris (1963)
Grothendieck, A.: Éléments de géométrie algébrique. IV. Étude locale des schémas et des morphismes de schémas IV. Inst. Hautes Études Sci. Publ. Math. 32, 361 (1967)
Groupes de monodromie en géométrie algébrique. I. Lecture Notes in Mathematics, vol. 288. Springer, Berlin (1972). Séminaire de Géométrie Algébrique du Bois-Marie 1967–1969 (SGA 7 I). Dirigé par A. Grothendieck. Avec la collaboration de M. Raynaud et D. S, Rim
Haastert, B.: Über Differentialoperatoren und D-Moduln in positiver Charakteristik. Manuscripta Math. 58(4), 385–415 (1987)
Kashiwara, M.: Vanishing cycle sheaves and holonomic systems of differential equations. In: Algebraic Geometry (Tokyo/Kyoto, 1982), vol. 1016 of Lecture Notes in Mathematics, pp. 134–142. Springer, Berlin (1983)
Katz, N.M.: \(p\)-adic properties of modular schemes and modular forms. In: Modular Functions of One Variable, III (Proceedings of International Summer School, University of Antwerp, Antwerp, 1972), pp. 69–190. Lecture Notes in Mathematics, vol. 350. Springer, Berlin (1973)
Lyubeznik, G.: \(F\)-modules: applications to local cohomology and \(D\)-modules in characteristic \(p>0\). J. Reine Angew. Math. 491, 65–130 (1997)
Malgrange, B.: Polynômes de Bernstein–Sato et cohomologie évanescente. Analysis and topology on singular spaces, II, III (Luminy, 1981), vol. 101 of Astérisque, pp. 243–267. Society of Mathematics, France, Paris (1983)
Milne, J.S.: Étale Cohomology, vol. 33 of Princeton Mathematical Series. Princeton University Press, Princeton, NJ (1980)
Mustaţă, M.: Bernstein–Sato polynomials in positive characteristic. J. Algebra 321(1), 128–151 (2009)
MacPherson, R., Vilonen, K.: Elementary construction of perverse sheaves. Invent. Math. 84(2), 403–435 (1986)
Verdier, J.-L.: Prolongement des faisceaux pervers monodromiques. Astérisque 130, 218–236 (1985). Differential systems and singularities (Luminy, 1983)
Acknowledgments
The author benefited from conversations with Sam Gunningham and was greatly assisted by notes from Kari Vilonen about the \(V\)-filtration in characteristic zero. The author would like to thank David Nadler for suggesting to pursue the \(V\)-filtration in positive characteristic in the crystalline setting and his patience and assistance while the author found the correct context for it. He is extremely grateful to Matthew Emerton for his assistance in leading the author through the unit \(F\)-module Riemann–Hilbert correspondence, the suggestion to consider tame ramification and supplying the author with very helpful insights into conducting research in positive characteristic geometry. Lastly, he would also like to thank the referee for providing many useful and thoughtful comments which undoubtedly improved the quality of this manuscript; particularly in the presentation of the proof of 4.2.1.
Author information
Authors and Affiliations
Corresponding author
Additional information
Author partially supported by NSF grant DMS-0636646.